耿美华/金治明
【内容提要】首先,针对一类线性倒向随机微分方程,给出了g-鞅同鞅之间相互联系所满足的充分条件。通过该条件得到了经典的Black-Scholes模型下未定权益的公平价格过程以及最优增长投资策略的价格过程。其次,引入了非惩罚的非线性倒向随机微分方程,并通过惩罚比率的不同取值来讨论相关的经济学意义。
【关 键 词】倒向随机微分方程/g-鞅/完备市场/套利
引言
Pardoux & Peng[1]证明了一类非线性倒向随机微分方程(BSDE)存在解,基于这样的BSDE,Peng[2]提出了g-期望和g-鞅的概念。由于g的非线性,使得g-期望是一类非线性数学期望。Peng[2],Chen and Peng[3],Briand,Coquet,Hu,MUmin and Peng[4]等人研究了某些g-期望和g-鞅的概念,如:g-鞅的Dood-Mayer分解定理,g-鞅的上穿不等式等。许多学者进一步研究倒向随机微分方程在数理金融、随机控制、偏微分方程、随机微分对微和经济等领域的应用,如El Karoui,Peng and Quenez[5],Chen and Kulperger[11],Peng and Yang[9]等。
本文通过建立特殊的g-鞅和经典鞅之间的联系,来进一步理解g-鞅的概念。将倒向随机微分方程的解(g-鞅)通过一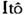
一、预备知识


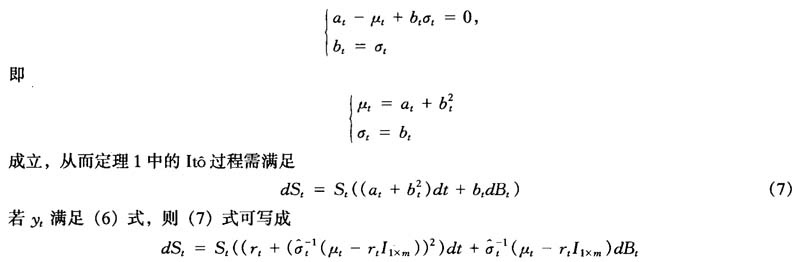
该过程同Platen[8]所给出的增长最优投资策略一致。注意到这里并没有用到增长最优投资策略的定义,但是通过其一个性质得到了价值过程。即,当市场存在等价鞅测度时,以增长最优投资策略为记帐单位,则投资策略的折现价格过程是客观概率下的鞅过程。
三、带惩罚的非线性倒向随机微分方程在金融市场中的应用
考虑如下形式的倒向随机微分方程


【参考文献】
[1]Pardoux, E. & Peng, S. Adapted solution of a backward stochastic differential equation[J]. Systems and Control Letters, 1990,14:55-61.
[2]Peng Shige. BSDE and related g-expectation[J]. Pitman Research Noted in Math. Series, 1996,364:141-160.
[3]Peng, S. Monotonic limit theorem of BSDE and nonlinear decomposition theorem of Doob-Mayer type[J]. Probab. Theory & Rel. Fields, 1999,113:473-499.
[4]Briand, P., Coquet, F., Hu, Y., Mémin, J., Peng, S. A converse comparison theorem for BDDEs and related properties of g-expectation[J]. Electron. Comm. Probab., 2000,5:101-117.
[5]ElKaroui, N., Peng, S. and Quenez, M. C. Backward stochastic differential equations in Finance[J]. Math, Finance, 1997,7:1-71.
[6]Chen Zengjing and Peng Shige. A general downcrossing inequality for g-martingales[J]. Statistics & Probability Letters, 2000,46:169-175.
[7]Peng Shige. Backward Stochastic Differential Equations, Nonlinear Expectation, Nonlinear Evaluations and Risk Measures[M]. Lecture Notes in Chinese Summer School in Mathematics, Weihai, 2004.
[8]Platen, E. Arbitrage in Continuous Complete Markets[J]. Adv. in Appl. Probab., 2002,34(3):540-558.
[9]Peng, S. and Yang, F. Duplicating and pricing contingent claims in incomplete markets[J]. Pacific Fconomic Review, 1999,4(3):237-260.
[10]金治明.数学金融学基础[M].北京:科学出版社,2006.
[11]Chen Zengjing and Reg Kulperger. Minimax pricing and choquet pricing[J]. Insurance Mathematics and Economics, 2006,38:518-528.^
转自《经济数学》(长沙)2009年1期第8~13页
【作者简介】耿美华,金治明,国防科技大学理学院(湖南长沙410073)
